"A Mathematical Theory of Communication" is an article by mathematician Claude E. Shannon published in Bell System Technical Journal in 1948.[1][2][3][4] It was renamed The Mathematical Theory of Communication in the 1949 book of the same name,[5] a small but significant title change after realizing the generality of this work. It has tens of thousands of citations which is rare for a scientific article and gave rise to the field of information theory. Scientific American referred to the paper as the "Magna Carta of the Information Age".[6]

Publication

The article was the founding work of the field of information theory. It was later published in 1949 as a book titled The Mathematical Theory of Communication (ISBN 0-252-72546-8), which was published as a paperback in 1963 (ISBN 0-252-72548-4). The book contains an additional article by Warren Weaver, providing an overview of the theory for a more general audience.

Contents

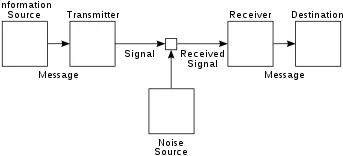
Shannon's diagram of a general communications system, showing the process by which a message sent becomes the message received (possibly corrupted by noise)

This work is known for introducing the contepts of channel capacity as well as the noisy channel coding theorem.

Shannon's article laid out the basic elements of communication:

  • An information source that produces a message
  • A transmitter that operates on the message to create a signal which can be sent through a channel
  • A channel, which is the medium over which the signal, carrying the information that composes the message, is sent
  • A receiver, which transforms the signal back into the message intended for delivery
  • A destination, which can be a person or a machine, for whom or which the message is intended

It also developed the concepts of information entropy and redundancy, and introduced the term bit (which Shannon credited to John Tukey) as a unit of information. It was also in this paper that the Shannon–Fano coding technique was proposed – a technique developed in conjunction with Robert Fano.

References

  1. ↑ Shannon, Claude Elwood (July 1948). "A Mathematical Theory of Communication" (PDF). Bell System Technical Journal. 27 (3): 379–423. doi:10.1002/j.1538-7305.1948.tb01338.x. hdl:11858/00-001M-0000-002C-4314-2. Archived from the original (PDF) on 1998-07-15. The choice of a logarithmic base corresponds to the choice of a unit for measuring information. If the base 2 is used the resulting units may be called binary digits, or more briefly bits, a word suggested by J. W. Tukey.
  2. ↑ Shannon, Claude Elwood (October 1948). "A Mathematical Theory of Communication". Bell System Technical Journal. 27 (4): 623–656. doi:10.1002/j.1538-7305.1948.tb00917.x. hdl:11858/00-001M-0000-002C-4314-2.
  3. ↑ Ash, Robert B. (1966). Information Theory: Tracts in Pure & Applied Mathematics. New York: John Wiley & Sons Inc. ISBN 0-470-03445-9.
  4. ↑ Yeung, Raymond W. (2008). "The Science of Information". Information Theory and Network Coding. Springer. pp. 1–4. doi:10.1007/978-0-387-79234-7_1. ISBN 978-0-387-79233-0.
  5. ↑ Shannon, Claude Elwood; Weaver, Warren (1949). The Mathematical Theory of Communication (PDF). University of Illinois Press. ISBN 0-252-72548-4. Archived from the original (PDF) on 1998-07-15.
  6. ↑ Goodman, Rob; Soni, Jimmy (2018). "Genius in Training". Alumni Association of the University of Michigan. Retrieved 2023-10-31.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.